What Is Continuity In Calculus And How To Calculate It?

Last Updated: 24 March 2023

Continuity is a key concept in mathematics and physics, and it’s important for understanding how functions work. In this section, we’ll explore what is continuity, the types of continuity, and how to calculate it.

Page Contents

What is Continuity?

In calculus, continuity refers to the continuous function. A function is continuous if it continues to be true as the input values become larger and larger.

Continuity is important for understanding how functions work. A function is continuous if there’s a smooth curve between all the points where the function is defined, called the graph of the function.

The derivative of a function at a point is continuous. If you take the derivative of a function at a point and use calculus to calculate how much it changes as you move around the point, then the derivative is always continuous.

This means that if you know what kind of derivative (linear, quadratic, etc.), then you can figure out whether or not a given function is continuous at any given point.

The inverse function of a function is also continuous. If you have an equation describing one side of a differential equation, then whichever side has the inverse function of a function on it will also be continuous.

In other words, if f(x) = g(y), then y–x = f(g(x)) should be continuous because f′(x) = g′(y). This rule usually applies only when both sides of an equation have derivatives.

Types of Continuity in Calculus

Continuity is a key concept in mathematics and physics, and it’s often used to describe the behavior of objects or systems. In this post, we’ll be discussing three main types of continuity:

Pointwise Continuity
Uniform Continuity

Absolute Continuity

1. Pointwise Continuity

When dealing with points in a domain, continuous functions are said to be continuous at each point in the domain. This means that the function is equal to its value at every point, no matter how close those points are to each other.

For example, consider the function f(x) which takes on the values 0 and 1 inside the interval [0 , 1]. The function is said to be continuous at x = 0 because it equals 1 there; it’s also continuous at x = 1 because that value is also an answer to f(x).

In other words, f(x) is continuously differentiable at x = 0 and x = 1.

2. Uniform Continuity

A function that’s continuous at all points in its domain but has a limit as x approaches some specific value is called uniform continuous (or simply uniform).

Uniform continuous functions satisfy two important requirements:

First, for any two points within the domain (regardless of their distance from each other), the limit must exist.
Second, if you know what that limit is then you can calculate how far away any given point lies from that limiting value.

For example, consider again our function f(x) when graphed on an axis representing the distance from zero (i.e., y-axis), we see that as x gets closer to zero – meaning closer towards the bottom left corner – f(x) starts taking on negative values (since -1 < x <= 1).

3. Absolute Continuity

Finally, let’s talk about absolute continuous functions: these are functions whose integrals over any closed interval can always be calculated using calculus methods such as limits or derivatives.

An example would be Newton’s Second Law of Motion which states that force equals mass times acceleration.

Steps of Continuity

There are three main methods or steps to calculate the continuity of the function.

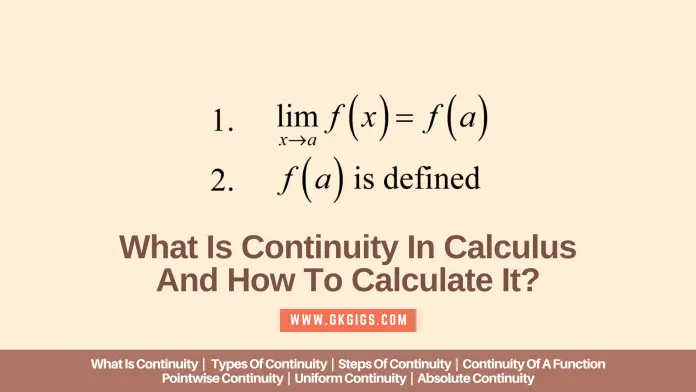
f(a) exists (function must be defined on “a”)
lim_x→a f(x) exists (limit of the function at “a” must exist)
f(a) = lim_x→af(x)

Let us briefly describe the above steps to understand how to calculate the continuity of a function.

1. f(a) exists

First of all, check whether the given function exists or not at the given point. For this, take the given function and put the value of the specific point in place of the independent variable of the function.

For Example:

If the given function f(x) = 3x³ + 12 and the specific point is 2. Then,

f(2) = 3(2)³ + 12 = 3(8) + 12 = 24 + 12 = 36

Hence the given function exists.

2. lim_x→a f(x) exists

After checking whether the given function exists or not, apply the limit to the given function that a function approaches. The limit of the function must exist at the given point.

For Example:

If the given function f(x) = 3x³ + 12 and the specific point is 2. Then,

lim_x→a f(x) = lim_x→2 [3(x)³ + 12]

lim_x→a f(x) = lim_x→2 [3(x)³] + lim_x→2 [12]

lim_x→a f(x) = [3(2)³] + [12] = 3(8) + 12 = 24 + 12 = 36

Hence the limit of the given function exists.

3. f(a) = lim_x→af(x)

The third and final step of checking the continuity of the function is to check whether the function at a specific point and the limit of that function has the same result or not. If the results are the same then the function is continuous otherwise it is discontinuous.

If the above three points hold then the function is said to be continuous. You can also try a Continuity Calculator for checking the continuity of the function according to the above three steps.

How to calculate the Continuity of a function?

Here are a few examples to learn how to calculate the continuity of a function.

a) Example 1:

Calculate the continuity of the given function at a specific point 3.

f(y) = 4y³ + 12y + 4

Solution:

Step 1: First of all, check whether the given function f(y) exists or not at y = 3.

f(y) = 4y³ + 12y + 4

f(3) = 4(3)³ + 12(3) + 4

f(3) = 4(27) + 12(3) + 4

f(3) = 108 + 36 + 4

f(3) = 148

Hence the given function exists at y = 3.

Step 2: Now check the limit of the function at 3.

Lim_y_→a f(y) = Lim_y_→3 [4y³ + 12y + 4]

Lim_y_→3 [4y³ + 12y + 4] = Lim_y_→3 [4y³] + Lim_y_→3 [12y] + Lim_y_→3 [4]

Lim_y_→3 [4y³ + 12y + 4] = 4(3)³ + 12(3) + 4

Lim_y_→3 [4y³ + 12y + 4] = 4(27) + 12(3) + 4

Lim_y_→3 [4y³ + 12y + 4] = 108 + 36 + 4 = 148

A limit of the given function exists.

Step 3: Now check whether the above two steps have a similar result or not.

f(y) = Lim_y_→a f(y)

148 = 148

Hence all three steps are satisfied. So the given function is continuous at 3.

b) Example 2

Calculate the continuity of the given function at a specific point 0.

f(z) = sin(z)/z

Solution:

Step 1: First of all, check whether the given function f(z) exists or not at z = 0.

f(z) = sin(z)/z

f(0) = sin(0)/0

f(0) = 0/0

Hence the given function is undefined at z = 0.

Step 2: Now check the limit of the function at 0.

Lim_z_→a f(z) = Lim_z_→0 [sin(z)/z]

Lim_z_→0 [sin(z)/z] = Lim_z_→0 [sin(z)] / Lim_z_→0 [z]

Lim_z_→0 [sin(z)/z] = [sin(0)] / [0] = 0/0

According to L’hopital’s rule of limit.

Lim_z_→0 [sin(z)/z] = Lim_z_→0 [d/dz sin(z)/d/dz (z)]

Lim_z_→0 [sin(z)/z] = Lim_z_→0 [cos(z) / (1)]

Lim_z_→0 [sin(z)/z] = Lim_z_→0 [cos(z)]

Lim_z_→0 [sin(z)/z] = cos(0)

Lim_z_→0 [sin(z)/z] = 1

A limit of the given function exists.

Step 3: Now check whether the above two steps have a similar result or not.

f(z) = Lim_z_→a f(z)

undefined = 1

Hence the third step is not satisfied. So the given function is discontinuous at 0.

c) Example 3

Calculate the continuity of the given function at a specific point 3.

f(w) = (4w³+ w² + 12) / (w + 2)

Solution:

Step 1: First of all, check whether the given function f(w) exists or not at w = 3.

f(w) = (4w³+ w² + 12) / (w + 2)

f(3) = (4(3)³+ 3² + 12) / (3 + 2)

f(3) = (4(27)+ 9 + 12) / (3 + 2)

f(3) = (108 + 9 + 12) / (3 + 2)

f(3) = (129) / (5)

f(3) = 25.8

Hence the given function is defined at w = 3.

Step 2: Now check the limit of the function at 3.

Lim_w_→a f(w) = Lim_w_→3 [4w³+ w² + 12) / (w + 2)]

Lim_w_→3 [4w³+ w² + 12) / (w + 2)]= (Lim_w_→3 [4w³]+ Lim_w_→3 [w²] + Lim_w_→3 [12]) / (Lim_w_→3 [w] + Lim_w_→3 [2])

Lim_w_→3 [4w³+ w² + 12) / (w + 2)] = (4(3)³+ 3² + 12) / (3 + 2)

Lim_w_→3 [4w³+ w² + 12) / (w + 2)] = (4(27)+ 9 + 12) / (3 + 2)

Lim_w_→3 [4w³+ w² + 12) / (w + 2)] = (108 + 9 + 12) / (3 + 2)

Lim_w_→3 [4w³+ w² + 12) / (w + 2)] = (129) / (5)

Lim_w_→3 [4w³+ w² + 12) / (w + 2)] = 28.5

A limit of the given function exists.

Step 3: Now check whether the above two steps have a similar result or not.

f(w) = Lim_w_→a f(w)

28.5 = 28.5

Hence all the steps are satisfied. So the given function is continuous at 3.

Wrap Up

In this section, we have covered all the basics of continuity in calculus along with its definition, types, steps, and solved examples. Now you can grab all the basics of this topic from the above post.

Read More